Bojan Vukasinovic								home page

Mode Shapes of a Drop in Forced Vibration

As the acceleration amplitude is increased, the drop undergoes several transitions to states of increasing spatio-temporal complexity. This begins with basic modes shape of the forced drop consisted of harmonic standing waves that are induced by the diaphragm motion. The high-frequency, low-amplitude forcing enables basic modes shapes up to the moderate wavenumbers. As the forcing amplitude is increased, basic mode shape undergoes instability at the contact line that results in the appearance of subharmonic azimuthal waves. A striking lattice mode emerges after the coupling of azimuthal subharmonic and existed harmonic motion, and subsequently evolves into a highly agitated mode that precedes the free-surface breakup. Following the breakdown of the lattice structure, the frequency of the most energetic mode is half the driving frequency. Therefore, it is argued that moderate-wavenumber forcing of the low-viscosity sessile drop exerts a free-surface dynamics that is a crossover from harmonic to parametrically-driven dynamics. The prolonged instatement of the dominant subharmonic wave-pattern is attributed to the very strong initial harmonic waves that are generated by motion of the supporting diaphragm.


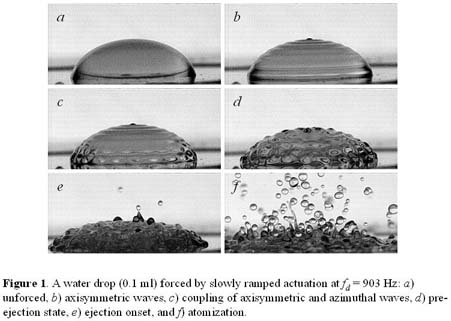
ramped forcing

Several stages in the behavior of a forced drop as the forcing amplitude is increased are shown in a sequence of side-view, high-speed images (500 fps) in Figures 1a-f. The unforced water drop (100 ml) is shown for reference in Figure 1a. Axisymmetric standing waves appeared on the free surface at low forcing amplitudes as shown in Figure 1b. This is a basic mode shape of oscillation for a supported drop. The mode shape shown in Figure 1b became visible at very low forcing amplitudes and had a frequency equal to the driving frequency (and some of its higher harmonics). At larger forcing amplitudes, an azimuthal instability of the basic mode shape appeared along the contact line of the drop, resulting in a loss of the axial symmetry of the free-surface shape (Figure 1c). Further increases in the forcing amplitude resulted in an increase in the amplitude and spatial complexity of the surface waves, as shown in Figure 1d. Eventually, the surface wave motion became intense enough to result in the ejection of secondary droplets from the wave crests (Figure 1e). It is noteworthy that when droplet ejection occurred, the amplitude of the wave motion on the entire free surface of the primary drop was approximately uniform and the ejection sites were uniformly distributed . Shortly after the onset of droplet ejection, the process accelerated rapidly and the primary drop completely atomized over a time interval of about 300 T (Figure 1f).


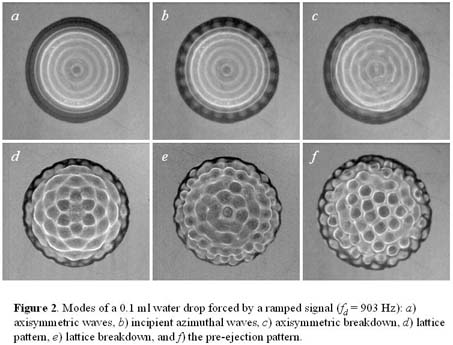
mode shapes


The resulting wave patterns are shown in a sequence of top-view, high-speed (1000 fps) video images in Figures 2a-f. The axisymmetric basic mode shape is shown in Figure 2a. The azimuthal instability was triggered along the contact line when the forcing amplitude was 18–22 g (Figure 2b). The azimuthal motion spread across the entire free surface of the drop as the forcing amplitude increased (Figure 2c). For forcing amplitudes near 34–38 g , the free surface wave pattern changed to one with a well-defined six-fold symmetry (Figure 2d). This wave pattern, termed a lattice pattern has five azimuthal rings at different radii with each ring shifted azimuthally by p/6 relative to the adjacent ring. When the forcing amplitude was 55–60 g, the sixfold symmetry of the waves in each ring appeared to be replaced by azimuthal waves with a higher wave number (Figure 2e). Finally, when the forcing amplitude was 130–135 g, the entire free surface was covered by a random distribution of wave cells that preceded droplet ejection (Figure 2f). The acceleration thresholds of the various instabilities increased with increasing driving frequency. It is noteworthy that the free surface (and internal) motions associated with these instabilities resulted in radial spreading of the primary drop. This effect was most pronounced at high forcing levels, as shown in Figures 2a and 2f, for example. For this case, the nominal diameter of the drop (as measured by the outer dark band) increased by 12%.

These results show that a forced sessile drop exhibits four distinct wave patterns, namely axisymmetric standing waves, azimuthal, lattice, and pre-ejection, each separated by a transition.

REFERENCES

											back to VIDA