Bojan Vukasinovic home page
When the acceleration of the diaphragm rises above a well-defined critical value, small secondary droplets begin to be ejected from the forced drop. Then, quite suddenly, the entire volume of the drop is ejected from the vibrating diaphragm in the form of a spray. This event is the result of an interaction between the fluid dynamical process of droplet ejection and the vibrational dynamics of the diaphragm. During droplet ejection, the effective mass of the drop-diaphragm system decreases and the resonance frequency of the system increases. If the initial forcing frequency is above the resonance frequency of the system, droplet ejection causes the system to move closer to resonance, which in turn causes more vigorous vibration and faster droplet ejection. This ultimately leads to drop bursting.

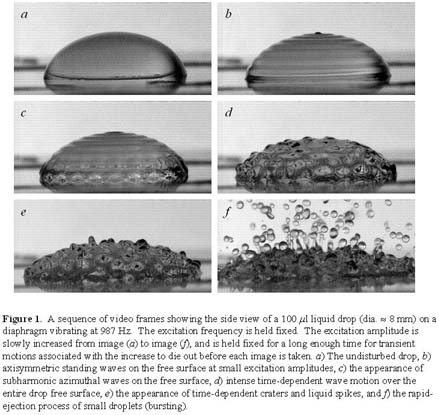
Figure 1 shows a sequence of video frames of a drop as the excitation amplitude is slowly increased and then held fixed for a long enough time for transient motions associated with the amplitude increase to die out. The frequency is fixed at 987 Hz. Figure 1a shows the unforced drop for reference. For small values of the excitation amplitude, axisymmetric standing waves exist on the free surface of the drop as shown in Figure 1b. These waves have the same frequency as the excitation and are present at even very small values of the excitation amplitude. Above a critical excitation amplitude, an azimuthal mode of instability is triggered along the contact line of the drop. This mode couples with the existing axisymmetric waves to produce an azimuthal, high-wave-number wave on the free surface of the drop (Figure 1c). This instability is also signaled by the appearance of a subharmonic frequency in the free-surface motion — the signature of a classic Faraday-wave instability. When the excitation amplitude is increased further, the free-surface waves increase in magnitude and complexity and become time dependent (Figure 1d). The development of distinct craters and liquid spikes that are in continual time-dependent motion are shown in Figure 1e. Finally, Figure 1f shows the initial phase of the bursting process.
The rate of droplet ejection depends on the excitation amplitude, but more interesting is the fact that for a fixed excitation amplitude the rate of droplet ejection may increase or decrease with time. When droplet ejection begins, wave motion and the droplet ejection sites seem to be evenly distributed over the entire free surface of the drop. The most interesting event, bursting, occurs at some finite time after the first appearance of droplet ejection. The length of this time interval depends on the excitation amplitude. When a large excitation signal is suddenly applied to the diaphragm, bursting occurs almost immediately. For smaller excitation amplitudes (but still large enough), bursting may be delayed on the order of seconds to maybe a minute or more after the forcing is applied. In some instances bursting does not occur at all, even though droplet ejection is present.
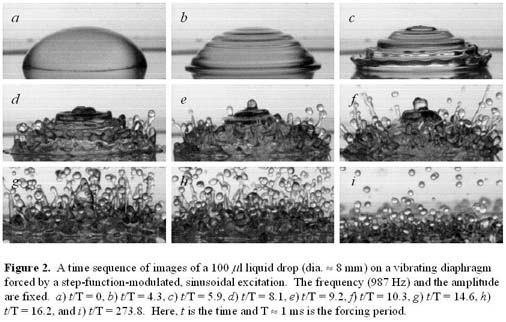
The sequence of images presented in Figure 2 shows what happens when a step-function-modulated sinusoidal excitation signal with a prescribed frequency and amplitude is applied to the diaphragm. Note that all of the different modes of instability on the drop free surface described in the previous case are still present. There is the initiation of axisymmetric waves in Figure 2b, the growth of the azimuthal instability in Figure 2c, and the development of complex wave patterns and bursting in the remaining images. The time from the initiation of the excitation until the first secondary droplets are ejected is about 8 forcing periods (Figure 2d). In real time, this is only 8 ms. Figure 2i is just about when the entire primary drop is completely atomized. For this case, the bursting event took about 0.3 seconds.
REFERENCES
back to VIDA